Assignment #8

Altitudes and Orthocenters

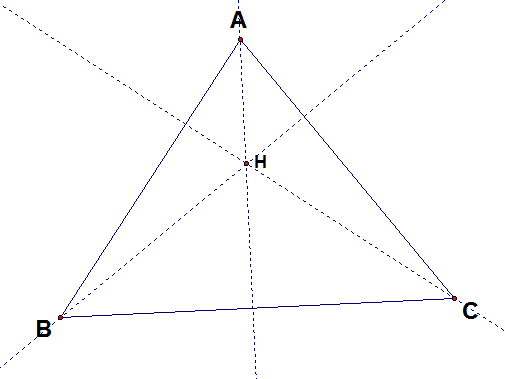
Let’s take a look at a triangle orthocenter. First we construct a triangle ABC. Then we create all three altitudes which have a common intersection at point H, the orthocenter.

Since ABC is an acute triangle, the orthocenter lies inside the triangle. We can also construct three additional triangles with the orthocenter H as one of the vertices.

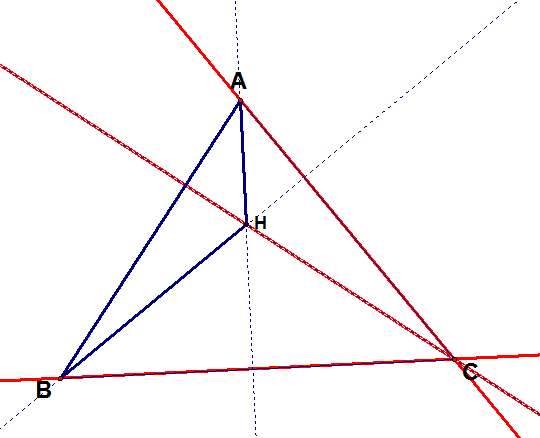
Let’s construct triangle HBC and show the altitudes.

Let’s also construct triangle HAB and show its altitudes.

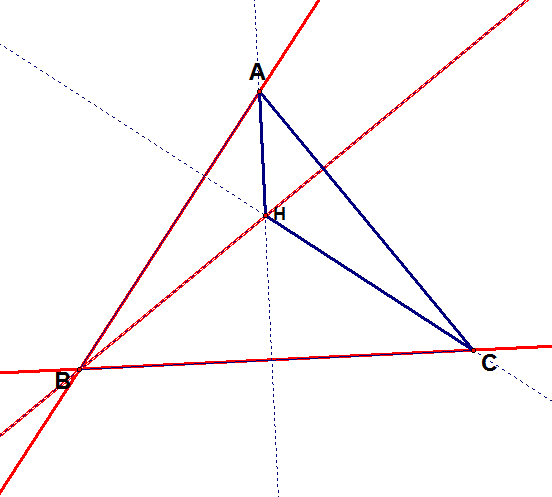
Finally, let’s construct triangle HAC and show its altitudes.

**pic4

All three triangles created with the orthocenter H and two vertices from the original triangle have similar properties, so we’ll use triangle HBC to make any conjectures and prove them.

Conjecture: The altitude of the triangle passing through the orthocenter H is the same as the altitude of the large triangle ABC passing through the vertex not shared with the smaller triangle HBC.

Proof: Since triangle ABC shares a common side with the smaller triangle HBC, and the orthocenter H lies along the altitude of triangle ABC, the altitude is the same.

Conjecture: The altitudes of the other two sides of the smaller triangle HBC contain the two non shared sides of the large triangle ABC.

Proof: Both sides of triangle HBC, one from point H to point B and the other from point H to point C, lie on the altitude of the side of the larger triangle opposite that vertex, so the sides of the small triangle HB and HC are perpendicular to the sides of the large triangle AC and AB respectively. The converse is also true: The side of the large triangle is perpendicular to the opposite side of the small triangle. The altitude of that small triangle side passes through the shared point C, so the altitude of the small side contains the side of the larger triangle.

Conjecture: Triangle HBC is obtuse whenever triangle ABC is acute and is acute whenever triangle ABC is obtuse.

Proof: From our work in Assignment #4, we found that the orthocenter lies inside the triangle when it is acute, it lies on the right angle vertex in a right triangle, and it lies outside the triangle when the triangle is obtuse. The altitudes from triangle HBC intersect at point A, so point A is the orthocenter of HBC.

Whenever point A is outside of triangle HBC, triangle ABC is acute and triangle HBC is obtuse. Whenever point A is inside triangle HBC, triangle ABC is obtuse and triangle HBC is acute.